Question

Question in image. Need help answering all 3 parts.

Answer 1

`~~~~~~~~~~~~ \textit{Amortized Loan Value} \\\\ pymt=P\left[ \cfrac{(r)/(n)}{1-\left( 1+ (r)/(n)\right)^(-nt)} \right]\implies pymt=P\left[ \cfrac{(r)/(n)}{1-\left((n)/(n+r)\right)^(nt)} \right]`

`~~~~~~ \begin{cases} P= \begin{array}{llll} \textit{original amount deposited}\\ \end{array}\dotfill & \begin{array}{llll} 48000 \end{array}\\ pymt=\textit{periodic payments}\\ r=rate\to 6.1\%\to (6.1)/(100)\dotfill &0.061\\ n= \begin{array}{llll} \textit{times it compounds per year}\\ \textit{monthly, thus twelve} \end{array}\dotfill &12\\ t=years\dotfill &6 \end{cases}`

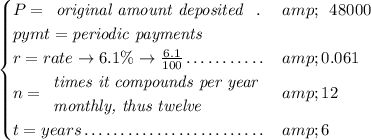
`pymt=48000\left[ \cfrac{(0.061)/(12)}{1-\left((12)/(12+0.061)\right)^(12 \cdot 6)} \right] \\\\\\ pymt=48000\left[ \cfrac{(61)/(12000)}{ ~~ (18249137)/(59666181) ~~ } \right] \implies {\Large \begin{array}{llll} pymt \approx 797.77 \end{array}}`

well, Linda is paying that much every month for 6 years or namely 72 months, so the total for those 72 months will simply be 72(797.77) ≈ 57439.44.

hmmmm what's the interest? well, if we simply subtract the principal from the total amount Linda is paying that'll be 57439.44 - 48000 ≈ 9439.44.