Question

Find the surface area of a cone with slant height 10 cm and diameter 12 cm to the nearest hundredth

Answer 1

Given:

Slant height = 10 cm

Diameter = 12 cm

Let's find the surface area of the cone.

To find the surface area of the cone, apply the formula:

`A=\pi rl+\pi r^2`

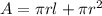
Where:

L is the slant height = 10 cm

r is the radius = diameter/2 = 12/2 = 6 cm

Plug in the values into the formula and solve for surface area A:

`\begin{gathered} A=(\pi *6*10)+(\pi *6^2) \\ \\ A=188.496+113.097 \\ \\ A=301.59\text{ cm}^2 \end{gathered}`

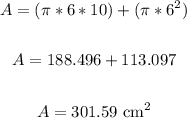
Therefore, the surface area of the cone to the nearest hundredth is 301.59 cm².

ANSWER:

301.59 cm²