Question

Sofia earned a score of 29 on Exam A that had a mean of 23 and a standarddeviation of 5. She is about to take Exam B that has a mean of 350 and astandard deviation of 20. How well must Sofia score on Exam B in order to doequivalently well as she did on Exam A? Assume that scores on each exam arenormally distributed.

Answer 1

Step 1: Write out the formula for finding the z-score of a number

`z=(x-\mu)/(\sigma)`
`\begin{gathered} z=\text{ the z-score} \\ x=\text{ the number whose z-score we are looking for} \\ \mu=\text{ the mean of the distribution} \\ \sigma=the\text{ standard deviation of the distribution} \end{gathered}`

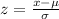
Step 2: Find the z-score of 29

`\begin{gathered} In\text{ this case,} \\ \mu=23,\sigma=5,x=29 \end{gathered}`

Therefore,

`z=(29-23)/(5)=(6)/(5)`

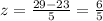
Step 3: Find a score on Exam B that has a z-score 6/5

`\begin{gathered} In\text{ this case,} \\ \mu=350,\sigma=20,x=\text{?} \end{gathered}`

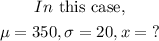
Therefore,

`(6)/(5)=(x-350)/(20)`

Cross-multiplying we have

`\begin{gathered} 6*20=5(x-350) \\ 120=5x-1750 \\ 120+1750=5x \\ 5x=1870 \\ x=(1870)/(5) \\ x=374 \end{gathered}`

Hence, Sofia must score 374 marks in Exam B in order to do equivalently well as she did on Exam A