Question

PLEASE HELP ME

Find the length of the third side. If necessary, round to the nearest tenth.
11 12

Answer 1

16.3

Explanation:

Use the Pythagorean theorem: a²+b²=c²

Solve this formula for c (the hypotenuse)

`c=\sqrt{a^(2)+b^(2) }`

then plug in a and b

`c=\sqrt{11^(2) + 12^(2) }`

`c=√(121+144)`

`c=√(265)`

Rounded to the nearest 10th you get 16.3

Answer 2

Answer: 16.3 units

Explanation:

We will use the Pythagorean theorem to help us solve because this is a right-triangle. a and b are the legs, and c is the hypotenuse. In this case, c is our unknown value.

Given:

a² + b² = c²

Substitute:

11² + 12² = c²

Square:

121 + 144 = c²

Add:

265 = c²

Square root both sides of the equation and reflexive property:

c =
`√(265)`

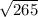
Square root:

c = 16.2788

Round to the nearest tenth:

c ≈ 16.3