To find all the zeros of a polynomial, we have to factor it completely. We'll have a series of factors in the form:

Were a is a zero of the polynomial.
Let's find the zeros of the given polynomial:
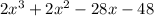
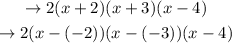
Thereby, the zeros of the polynomial are -2, -3 and 4. Each one with a multiplicity of one (They appear only one time)