Question

Determine whether each sequence is an arithmetic sequence. If so, find the common difference and the next three terms. 1. -1, 2, -3, -4, ... 2. 1.25, 3.75, 6.25, 8.75, ...

Answer 1

1. it is not an arithmetic sequence

2.arithmetic sequence

`\begin{gathered} \text{term}_5=11.25 \\ \text{term}_6=13.75 \\ \text{term}_7=16.25 \end{gathered}`

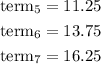
Step-by-step explanation

Step 1

find the common difference for the sequence.

`\begin{gathered} -1,2,-3,4 \\ \text{term}_1=-1 \\ \text{term}_2=2 \\ \text{term}_3=-3 \\ term_4=-4 \\ term2-term1=2-(-1)=2+2=4 \\ \text{term}3-\text{term}2=-3-2=-5 \\ \text{term}4-\text{term}3=-4-(-3)=-4+3=-1 \end{gathered}`

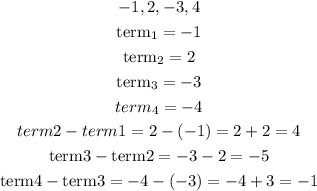
there is no common difference , so this is not an arithmetic sequence

Step 2

2.

find the common difference

`\begin{gathered} 1.25,3.75,6.25,8,75 \\ \text{term}1=1.25 \\ \text{term}2=3.75 \\ \text{term}3=6.25 \\ \text{term}4=8.75 \\ \text{differences} \\ \text{term}2-\text{term}1=3.75-1.25=2.5 \\ \text{term}3-\text{term}2=6.25-3.75=2.5 \\ \text{term}4-\text{term}2=8.75-6.25=2.5 \end{gathered}`

so, the common difference is 2.5, in other words, it means you have to add 2.5 to obtain the next term

Step 2.1

now, let's find the next three terms

`\begin{gathered} \text{rule} \\ \text{term}_(n+1)=term_(n+2.5) \\ \text{then} \\ \text{term}5=\text{term}4+2.5 \\ \text{term}5=8.75+2.5=11.25 \\ term6=11.25+2.5=13.75 \\ \text{term}7=13.75+2.5=16.25 \end{gathered}`

I hope this helps you