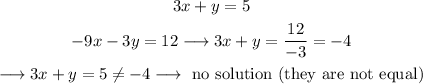Question

6. 5. Convert each function to slope-intercept form, and then determine in which quadrant the solution falls by graphing the following system of equations. 3x + y = 5 slope-intercept = -9x-3y = 12 slope- intercept = In Ou

Answer 1

The slope intercept form of a line is:

`y=mx+b`

Then we can write each of the equations as:

`\begin{gathered} 3x+y=5 \\ y=5-3x \\ y=-3x+5 \end{gathered}`
`\begin{gathered} -9x-3y=12 \\ -3y=12+9x \\ y=(12)/(-3)+(9x)/(-3) \\ y=-4-3x \\ y=-3x-4 \end{gathered}`

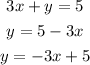
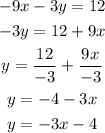
We have parallel lines, as they both have the same slope (m=-3).

If we graph the lines, we get:

The lines don't intersect, so we have no solution.

We can demonstrate this as:

`\begin{gathered} 3x+y=5 \\ -9x-3y=12\longrightarrow3x+y=(12)/(-3)=-4 \\ \longrightarrow3x+y=5\\e-4\longrightarrow\text{ no solution (they are not equal)} \end{gathered}`