Question

which has the larger 15th term when comparing the arithmetric and geometric sequences below? show evidence that support your answer Arithmetic sequence: 150, 650, 1150, 1650Geometric sequence:4,12, 36, 108

Answer 1

For the arithmetic sequence,

15th term = 7,500

For the geometric sequence,

15th term = 19,131,876

We can see that the geometric sequence has the larger 15th term.

Step-by-step explanation

The general formula for an arithmetic progression is

f(n) = a + (n - 1)d

where

a = first term = 150

n = number of terms

d = common difference

= (Second term) - (First term)

= (Third term) - (Second term)

= Difference between consecutive terms

= 650 - 150

= 500

f(n) = 150 + (n - 1)500

f(n) = 150 + 500n - 500

f(n) = -350 + 500n

For the 15th term, n = 15

f(n) = -350 + 500n

f(15) = -350 + 500(15)

f(15) = -350 + 7500

f(15) = 7,150

For the geometric sequence,

`f(n)=ar^(n-1)`

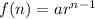
where

a = first term = 4

n = number of terms

r = common ratio

= (Second term)/(First term)

= (Third term)/(Second term)

= Ratio of consecutive terms

= (12/4)

= 3

For the 15th term, n = 15

`\begin{gathered} f(n)=ar^(n-1) \\ f(15)=4*3^(15-1) \\ f(15)=4*3^(14) \\ f(15)=19,131,876 \end{gathered}`

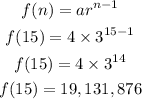
Hope this Helps!!!