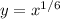Question

Which properties would be used to solve the logarithmic expression

Answer 1

C. Power rule

E. Equality rule

Step-by-step explanation

The power rule of logarithms is:

`\log a^b=b\log a`

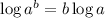
It can be applied in both ways. This means that in this expression we can put the power back:

`\begin{gathered} 3\log y=(1)/(2)\log x \\ \log y^3=\log x^(1/2) \end{gathered}`

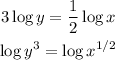
So first we would use the power rule.

Then, we have log on both sides. The equality rule is:

`\begin{gathered} \text{if }\log a=\log b \\ \text{then }a=b \end{gathered}`

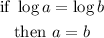
So for this expression:

`\begin{gathered} \log y^3=\log x^(1/2) \\ y^3=x^(1/2) \end{gathered}`

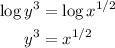
So next we would apply the equality rule.

And then we would finish solving the equation:

`y=x^(1/6)`