Question

Determine the value of m so that (x-2) is a factor of x^3 + 2mx^2 +6x -4.

Answer 1

Answer:

m = -2

Step-by-step explanation:

The given function is:

`f(x)=x^3+2mx^2+6x-4`

For x - 2 to be a factor of f(x):

f(x) must be equal to zero when x = 2

`\begin{gathered} f(2)=2^3+2m(2^2)+6(2)-4 \\ f(2)=8+8m+12-4 \\ f(2)=8m+16 \end{gathered}`

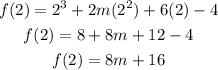
Let f(2) = 0 to solve for m

8m + 16 = 0

8m = -16

m = -16/8

m = -2

Therefore, for x - 2 to be a factor of x^3 + 2mx^2 +6x -4, the value of m is -2