Question

There are 150 adults and 225 children at a zoo. If the zoo makes a total of $5100 from the entrance fees, and of an adult and a child to attend is $31, how much does it cost for a parent and child

Answer 1

y+x=31, 150x+225y=5100.
y=-x+31
150x+225(-x+31)=5100
-75x=-1875
x=25 (adult tickets are $25)
31-25= 6 (child tickets are $6)

Answer 2

The entrance fee per parent is $25, while the cost per child is $6.

Explanation:

This problem is solved by using a system of equations.

We know that there are 150 adults and 225 children, which makes a total of $5100. So, the variables here will represent the cost per adult and the cost per children. The first equation is

`150x+225y=5100`

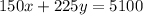
Where
`x` represents the cost per adult and
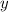`y` represents the cost per child.

We also know that the entrance fee of an adult and a child is $31, this means

`x+y=31`

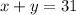
Now, we isolate
`x` in the second equation, to replace it into the first equation and solve for
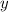`y`, as follows

`x+y=31\\x=31-y`

Then,

`150x+225y=5100\\150(31-y)+225y=5100\\4650-150y+225y=5100\\75y=5100-4650\\y=(450)/(75)=6`

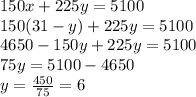
Now, we use this value to find the other one

`x+y=31\\x+6=31\\x=31-6=25`

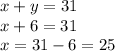
Therefore, the entrance fee per parent is $25, while the cost per child is $6.