Question

A wooden box weighing 150 N is sliding at a constant rate over a concrete sidewalk. The force required to pull the box at a steady rate is 78 N. What is the coefficient of friction?

1.9
228
72
0.52

Answer 1

78 also then equals the force of friction (steady rate, so sum of F = 0) Ffr = mu*Fn. Normal force = weight for this box = 150. mu = 78/150 = 0.52

Answer 2

0.52

Step-by-step explanation:

The box is moving at constant velocity along the horizontal direction: this means that its acceleration is zero. According to Newton's second law:

`F_(net)=ma=0`

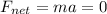
where
`F_(net)` is the net force acting on the object, m its mass, and a its acceleration, since the acceleration is zero, then the net force must be zero as well.

There are in total 4 forces acting on the box:

- along the vertical direction: the weight of the box (downward), given by
`W=150 N`, and the normal reaction of the floor on the box (upward),
`N`

- along the horizontal direction: the force that pulls the box,
`F=78 N`, and the frictional force, which is given by
`\mu N`, in the opposite direction

Since the net force is zero, the resultant on each direction must be zero. In the vertical direction we have:

`W-N=0\\N=W=150 N`

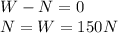
While in the horizontal direction we have

`F-\mu N=0\\\mu=(F)/(N)=(78 N)/(150 N)=0.52`

So, the coefficient of friction is 0.52.