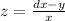Question

Solve for z. zx+y=dx

Answer 1

According to the given data we have the following equation:

zx+y=dx

To solve for the variable z the equation we would make the following steps:

`\begin{gathered} \text{First, }\mathrm{Subtract\: }y\mathrm{\: from\: both\: sides} \\ zx+y-y=dx-y \end{gathered}`

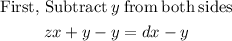
Next we would have to simplify the equation above, so:

`\begin{gathered} zx+y-y=dx-y \\ zx=dx-y \end{gathered}`
`\begin{gathered} \text{Next, }\mathrm{Divide\: both\: sides\: by\: }x;\quad \: x\\e\: 0 \\ (zx)/(x)=(dx)/(x)-(y)/(x);\quad \: x\\e\: 0 \end{gathered}`

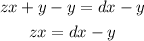
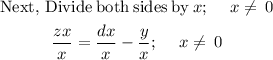
Therefore, the final result by dividing bith sides by x we would get the following for z:

`z=(dx-y)/(x)`