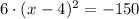Question

What is the intermediate step in the form (x+a)^2=b(x+a) 2 =b as a result of completing the square for the following equation?

Answer 1

For this problem, we are asked to provide the intermediate step we obtain while completing the square for the following expression:

`-6x^2-235=-48x+11`

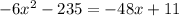
Completing the square means to transform the function into a expression such as:

`(a+b)^2=a^2+2\cdot a\cdot b+b^2`

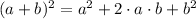
For this we will first change all the terms to the left side, as shown below:

`\begin{gathered} 6x^2-48x+235+11=0 \\ 6x^2-48x+246=0 \end{gathered}`

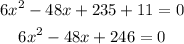
Since all three terms are divisible by 6, we need to use factorization to isolate the 6 from the equation:

`6\cdot(x^2-8x+41)=0`

Now, we need to rewrite the expression inside the parenthesis, such as we will obtain a form that is roughly equal to the perfect square we're looking for.

`\begin{gathered} 6\cdot(x^2-2\cdot4\cdot x+41)=0 \\ 6\cdot(x^2-2\cdot4\cdot x+16+25)=0 \end{gathered}`

Now, we need to remove the "25" from the parenthesis, for that we need to multiply the 6 by 25.

`\begin{gathered} 6\cdot(x^2-2\cdot4\cdot x+16)+6\cdot25=0 \\ 6\cdot(x^2-2\cdot4\cdot x+16)+150=0 \\ 6\cdot(x^2-2\cdot4\cdot x+16)=-150 \end{gathered}`

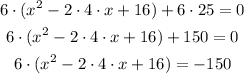
Now we can transform the parenthesis to the sum of two squares, where the term "a" is equal to x, and the "b" is equal to 4.

`6\cdot(x^{}-4)^2=-150`