Question

At what depth is there only one percent of surface sunlight? Do not round until the final answer the round to the nearest whole number as needed

Answer 1

From the question, we have been given the function as

`\begin{gathered} f(x)=20(0.995)^x \\ where\text{ f\lparen x\rparen represents percent sunlight } \\ x\text{ distance in feet } \end{gathered}`

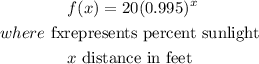
To solve this we substitute 1 for f(x) and solve the equation, we have

`\begin{gathered} f(x)=20(0.995)^x \\ 1=20(0.995)^x \\ 0.995^x=(1)/(20) \end{gathered}`

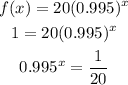
Taking log of both sides

`\begin{gathered} log(0.995)^x=log((1)/(20)) \\ xlog0.995=log((1)/(20)) \\ x=(log((1)/(20)))/(log0.995) \\ x=597.64733 \end{gathered}`

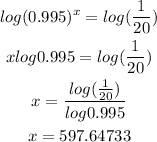
Hence the answer is 598 feet to the nearest whole number

This is shown on the graph as follows

The point (598, 1)