Question

The population for Bacteria A can be modeled by the function f(t)=5×4^t. The population for Bacteria B can be modeled by the function g(t)=10×2^t. For both functions, t is time in days. Which statement describes the difference in the populations after 4 days?A. The populations are the same.B. The population for Bacteria A is 2 times greater than that of B.C. The population for Bacteria B is 2 times greater than that of A.D. The population for Bacteria A is 8 times greater than that of B.E. The population for Bacteria B is 8 times greater than that of A.

Answer 1

The difference in population of Bacteria A and Bacteria B after 4 days is what we are after.

Population of Bacteria A is modelled as follows

`\begin{gathered} f(t)=5*4^t \\ \text{where t = time in days} \\ f(t)=5*4^4 \\ f(t)=5*\text{ }256 \\ f(t)=1280 \end{gathered}`

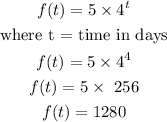
Population of Bacteria B is modelled as follows

`\begin{gathered} g(t)=10*2^t \\ g(t)=10*2^4 \\ g(t)=10*16 \\ g(t)=160 \end{gathered}`

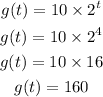
The answer is D . The population of bacteria A is 8 times greater than bacteria B.