Question

The third term of the sequence is 120. The fifth term is 76.8 The explicit rule for the geometric sequence is an=?(?)n-1

Answer 1

The explicit rule of the geometric sequence

aₙ = 187.5 (0.8)ⁿ⁻¹

Explanation:

Step(i):-

Given that the third term of the sequence = 120

tₙ = a rⁿ⁻¹

t₃ = a r³⁻¹ = ar²

120 = ar² ..(i)

Given that the fifth term of the given geometric sequence = 76.8

tₙ = a rⁿ⁻¹

t₅ = a r⁵⁻¹ = a r⁴

76.8 = a r⁴...(ii)

Step(ii):-

Dividing (ii) and (i)

`(ar^(4) )/(ar^(2) ) = (76.8)/(120)`

r² = 0.64

r =√ 0.64 = 0.8

Substitute r= 0.8 in equation (i)

120 = ar²

120 = a(0.8)²

⇒
`a = (120)/(0.64) =187.5`

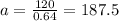
Step(iii):-

The explicit rule of the geometric sequence

aₙ = a rⁿ⁻¹

put a= 187.5 and r = 0.8

aₙ = 187.5 (0.8)ⁿ⁻¹