Question

14. The angle of elevation from a person lying on the ground to a hot-air balloon is 43 degrees The balloon is at an altitude of 1500 feet. To the nearest foot, find the horizontal distance from the person to a point on the ground directly below the balloon.

Answer 1

1609 ft

Step-by-step explanation:

First, we place the given angle in the diagram.

Since it is an angle of elevation, it means the person is looking upwards and the angle will be at point C as shown in the diagram below:

Since we are to determine the horizontal distance from the person to a point on the ground directly below the balloon, it means we are to find the distance BC in the diagram.

By trigonometric ratios:

`\begin{gathered} \tan \text{ C }=(AB)/(BC) \\ \tan 43^0=(1500)/(BC) \\ \text{Cross multiply} \\ BC*\tan 43^0=1500 \\ \text{Divide both sides by }\tan 43^0 \\ BC=(1500)/(\tan 43^0) \\ BC=1608.55\text{ ft} \end{gathered}`

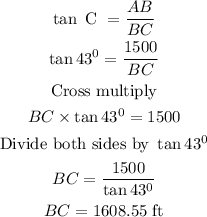
Therefore, to the nearest foot:

Horizontal Distance = 1609 ft.