The speed of a parachutitst in free fall is 65m/s. In order to find this velocity in feet per second you use the following identities:
1 m = 100 cm
2.54 cm = 1 in
12 in = 1 ft
Each of the previous identity is used as a convertion factor. You proceed as follow:
![65(m)/(s)\cdot\frac{100\operatorname{cm}}{1m}\cdot\frac{1in}{2.54\operatorname{cm}}\cdot(1ft)/(12in)=213.2(ft)/(s)]()
where you put as the denominator the quantity with the unit that it is necessay to cancel out. For instance, in the first factor (65m/s) you have meters in the numerator, in order to cancel meter, the next factor (100cm/1m) has to have a denominator with units in meter. Then you have a numerator with centimeters, and you use a factor that allows you to cancel centimeter, as the factor 1in/2.54cm makes.
To calculate the height traveled by the parachutist you use the following formula:
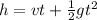
v: speed of the parachutist = 213.2 ft/s
t: time = 20 s
g: gravitational acceleration = 32ft/s²
You replace the previous values into the formula for h, the height traveled:
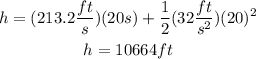
Hence, the parachutist falls 10664 ft