Question

A pizza shop sells pizza by the slice. They make two different sizes of pizza - a 14 -inch pizza which they cut into pi/3 radian slices and a 16-inch pizza which they cut into pi/4 radian slices. They sell a slice of each one for the same price. Which is a better deal? Show your work.drawing a diagram will help

Answer 1

A 14-inch pizza which they cut into pi/3 radian slices

Step-by-step explanation:

Note that the size of a pizza is its diameter.

Radius = Diameter/2

Case 1

A 14-inch pizza which they cut into pi/3 radian slices

Radius = 14/2 = 7 inches

`\begin{gathered} \text{Area of one slice}=((\pi)/(3))/(2\pi)*\pi*7^2 \\ =(\pi)/(6\pi)*\pi*7^2 \\ =(49\pi)/(6) \\ \approx8.2\pi\text{ square inches} \end{gathered}`

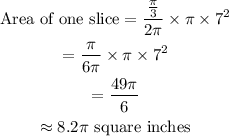
Case 2

A 16 -inch pizza which they cut into pi/4 radian slices

Radius = 16/2 = 8 inches

`\begin{gathered} \text{Area of one slice}=((\pi)/(4))/(2\pi)*\pi*8^2 \\ =(\pi)/(8\pi)*\pi*8^2 \\ =(64\pi)/(8) \\ =8\pi\text{ square inches} \end{gathered}`

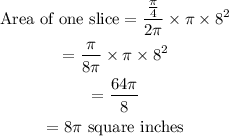
Since 8.2π square inches is greater than 8π square inches, the first case (a 14-inch pizza which they cut into pi/3 radian slices) is a better deal.