Answer:
+5300 kg m/s
Step-by-step explanation:
In any type of collision, the total momentum is conserved. Therefore, we can just calculate the total momentum before the collision, and the final momentum will be equal to the initial one.
The total momentum before the collision is:

where
 is the mass of the bus
is the mass of the bus
 is the mass of the car
is the mass of the car
 is the initial velocity of the bus
is the initial velocity of the bus
 is the initial velocity of the car
is the initial velocity of the car
Substituting the numbers, we find
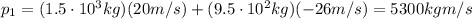
And since the total momentum is conserved, this is also the final momentum after the collision.