Question

The coordinates of quadrilateral FGHJ are F(-5, -3), G(-3, 3), H(3, 5), and J(7, -1). Find the midpoint of FGa- 0,4b- 1,-2c- 5,2d- -4,0

Answer 1

In this case, since we have to find the midpoint of FG, we only need to focus on that segment. In order to find the midpoint of a line that focuses through the points (x1,y1) and (x2,y2), we can apply the formula:

`\begin{gathered} xm=(x1+x2)/(2) \\ ym=(y1+y2)/(2) \end{gathered}`

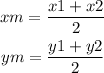
Where xm is the x-coordinate and ym the y-coordinate of the midpoint (xm,my), in this case the points of the segment FG are (-5,-3) and (-3,3), if we replace s their values into the above equation, we get:

`\begin{gathered} xm=(-5+(-3))/(2)=(-5-3)/(2)=(-8)/(2)=-4 \\ ym=(-3+3)/(2)=(0)/(2)=0 \end{gathered}`

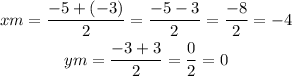
Then, the midpoint of FG is (-4,0)