Answer:
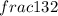
Explanation:
Half life is the time taken to reduce the original concentration of the reactants to half.
Now, the number of half lives is related to the amount left after decomposition by the formula:
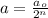
where,
a = amount of reactant left after n-half lives = ?
 = Initial amount of the reactant
= Initial amount of the reactant
n = number of half lives = 5
Putting values in above equation, we get:
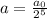

Thus the amount of sample that remains after 5 half lives is
 of original amount.
of original amount.