gDensity is a property of matter that relates the mass to its volume, we can calculate it with the following equation:
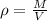
Where,

Now, we have to calculate the volume of the solid. We do that by calculating the volume of a cylinder. The equation is:

Where,
Pi=3.14
r= Radius = Diameter/2 = 9.5mm/2=4.75mm =0.475cm
h = Length = 20.8mm = 2.08cm
We replace the values:
![\begin{gathered} V=\pi*(0.475\operatorname{cm})^2*2.08\operatorname{cm} \\ V=1.47\operatorname{cm}^3 \end{gathered}]()
Now, we replace the known values in the density equation:
![\rho=(M)/(V)=\frac{19.8g}{1.47\operatorname{cm}^3}=13.4(g)/(cm^3)]()
So, the density of a cylindrical solid with a mass of 19.8g is 13.4g