We are given that 221J of work is required to lift a mass of 4.75 meters. The amount of work is determined by the following equation:

Where:
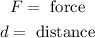
The force is equivalent to the combined weight if the dog and the basket multiplied by the acceleration of gravity:
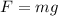
The distance is the height that the basket is lifted:

Now, we substitute in the equation:
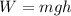
Now, we divide by "gh":
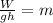
Now, we substitute the values:
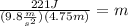
Solving the operations:
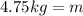
Therefore, the combined mass is 4.75 kg.