Question

You want to buy a 182,000 home. You plan to pay 15% as a down payment , and take out a 30 year loan for the rest .

Answer 1

Given:

There are given the total amount to buy a home $182000.

Step-by-step explanation:

From the given question, there are saying that the 15% as a down payment

Then,

The total amount that pays as a down payment.

So,

`\begin{gathered} 182000*15\%=182000*0.15 \\ =27300 \end{gathered}`

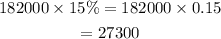
Then,

Subtract the above amount from the total amount:

So,

`182000-27300=154700`

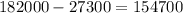
(a):

The total loan amount is $154700.

(b):

The total loan amount is 182000.

The total number of periods is 30.

The interest rate per period is 5%.

So,

For calculating the monthly payment:

Divide the given rate by 12 and multiply the given period by 12.

So,

`\begin{gathered} r=5\%=0.05 \\ =(0.05)/(12) \\ =0.0041 \\ n=30 \\ =30*12 \\ =360 \end{gathered}`

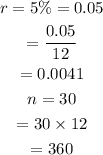
Now,

From the formula:

`\begin{gathered} A=(P(r(1+r)^n)/(((1+r)^n-1)) \\ A=(154700(0.0041(1+0.0041)^(360))/(((1+0.0041)^(360)-1)) \\ A=802.85 \end{gathered}`

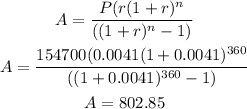
Then,

Put the all values into the above formula:

So,

The monthly payment is $802.85.

Now,

(c):

If the interest rate is 6%:

Then,

`\begin{gathered} A=(P(r(1+r)^n))/(((1+r)^n-1)) \\ A=(154700(0.005(1+0.005)^(360)))/(((1+0.005)^(360)-1)) \\ A=(154700(0.005(1.005)^(360)))/(((1.005)^(360)-1)) \\ A=(4658.46)/(5.022) \\ A=927.61 \end{gathered}`

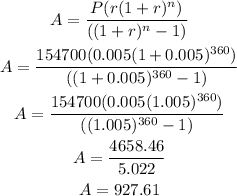
Final answer:

Hence, the answer of part (a), (b), and (c) are :

(a): $154700.

(b): $802.85

(c): $927.61