Question

Write the equation of the parabola in vertex form with the following conditions:Vertex: (0,4)Directrix: y = 2

Answer 1

We are given the vertex of the parabola (0,4) and the directrix y=2. This is a parabola with its axis of symmetry parallel to the y-axis.

The standard form of the parabola is

`\mleft(x-h\mright)^2=4p(y-k)`

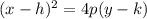
Where (h,k) is the vertex of the parabola and the directrix is given as

y = k - p

We can find the value of p:

p= k - y = 4 - 2 = 2

Substituting, we have the equation of the parabola:

`\begin{gathered} (x-0)^2=4\cdot2(y-4) \\ x^2=8(y-4) \end{gathered}`

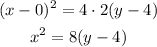
The first choice is correct