The given function
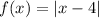
Represents a function for the absolute value of x, this indicates that the function is V shaped.
To graph it you have to determine at least three points: The vertex and one point for each arm of the function.
The coefficient that multiplies the absolute value is positive a=+1, this indicates that the V will open upwards.
The function is written in vertex form, if you remember the structure of this form:
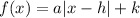
Where
h is the x-coordinate of the vertex → in the formula it always appears with the opposite sign. If you see it +, then the coordinate is negative and vice versa.
k is the y-coordinate of the vertex
For our function
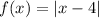
The value inside the absolute term is the x-coordinate of the vertex: h=+4
There is no value outside the absolute term, this indicates that the y-coordinate of the vertex is zero: k=0
Vertex: (4, 0)
Now we have to determine one point for the left arm of the function and one value for the right arm of the function.
Since the vertex is at x=4, I'll use x=2 for the left point and x=6 for the right point
Left point
Replace the formula with x=2
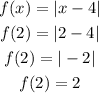
The left point is (2, 2)
Right point
Replace the formula with x=6
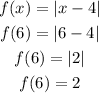
The right point is (6, 2)
Now using the three points you can plot the function.