ANSWER
The value of x is 4
EXPLANATIONS;
Given that
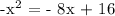
Re-write the quadratic function
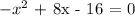
Recall, that the general form of quadratic function is given as
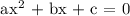
Relating the two functions together
a = -1
b = 8
c = - 16
Determine the number of solutions first using the discriminant
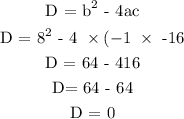
Since D = 0 , then , the quadratic function has one real solution
Solve the equation using the general quadratic formula

Hence, the value of x is 4