Question

Complete the measure of the interior angles of the given triangle.

Answer 1

It says that the given triangle is an isosceles triangle, which means that two of its sides are congruent.

As shown in the figure, the sides across ∠A and ∠C are congruent. This condition must also mean that ∠A and ∠C are congruent.

`\angle A\text{ = }\angle C`

∠C and its adjacent angle measuring 130° are Supplementary, which means that their sum is equal to 180°.

With this, we can get the measure of ∠C.

`\begin{gathered} \angle C+130^(\circ)=180^(\circ) \\ \angle C+130^(\circ)-130^(\circ)=180^(\circ)-130^(\circ) \\ \angle C=180^(\circ)-130^(\circ) \\ \angle C=50^(\circ) \end{gathered}`

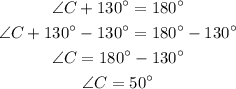
But,

`\angle A\text{ = }\angle C`

Therefore, ∠A must also be equal to 50°.

`\angle A\text{ = }\angle C=50^(\circ)`

The total sum of all interior angles of a triangle is 180°. With that relationship, we can determine the measure of ∠B since we've already determined the measure of ∠A and ∠C.

We get,

`\angle A\text{ + }\angle B\text{ + }\angle C=180^(\circ)`
`\begin{gathered} \text{ 50}^(\circ)\text{ + }\angle B+50^(\circ)=180^(\circ) \\ \angle B+100^(\circ)-100^(\circ)=180^(\circ)-100^(\circ) \end{gathered}`
`\angle B=80^(\circ)`

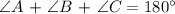
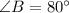
Therefore, ∠A = 50°, ∠B = 50° and ∠C = 80°.