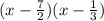Question

factor each trinomial if the trinomial cannot be factored write prime. show ALL work1.) 2x^2+5x-122.) 6x^2-23x+7

Answer 1

1) the initial expression is:

`2x^2+5x-12`

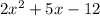
To factor this expression we can use the cuadratic formula that is:

`\begin{gathered} x=\frac{-b\pm\sqrt[]{b^2-4(a)(c)}}{2(a)} \\ a=2 \\ b=5 \\ c=-12 \end{gathered}`

so we replace the values and we get:

`\begin{gathered} x=\frac{-5\pm\sqrt[]{5^2-4(2)(-12)}}{2\cdot2} \\ x=\frac{-5\pm\sqrt[]{121}}{4} \\ x=(-5\pm11)/(4) \end{gathered}`

So now we have two solutions so:

`\begin{gathered} x_1=(-5+11)/(4)=(6)/(4)=(3)/(2)=1.5 \\ x_2=(-5-11)/(4)=-(16)/(4)=-4 \end{gathered}`

so the factor expression will be:

`(x-(3)/(2))(x+4)`

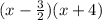
2) the initial expression is:

`6x^2-23x+7`

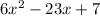
so we repeat the same procedure so:

`\begin{gathered} x=\frac{23\pm\sqrt[]{23^2-4(6)(7)}}{2(6)} \\ x=\frac{23\pm\sqrt[]{529-168}}{12} \\ x=\frac{23\pm\sqrt[]{361}}{12} \\ x=(23\pm19)/(12) \end{gathered}`

So the two possible solutions are:

`\begin{gathered} x_1=(23+19)/(12)=(42)/(12)=(7)/(2)=3.5 \\ x_2=(23-19)/(12)=(4)/(12)=(2)/(6)=(1)/(3)=0.33 \end{gathered}`

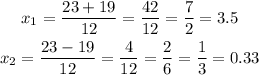
so the factor form is:

`(x-(7)/(2))(x-(1)/(3))`