Question

Find the average rate of change of the given function on the interval [1,5] g(x)=-3x^2+x+1

Answer 1

Answer: -17

=====================================================

Step-by-step explanation:

Plug in x = 1 which is the left endpoint of the interval [1,5] aka
`1 \le \text{x} \le 5`

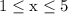
g(x)=-3x^2+x+1

g(1)=-3(1)^2+1+1

g(1) = -1

Then plug in x = 5 which is the right endpoint of that interval mentioned.

g(x) = -3x^2+x+1

g(5) = -3(5)^2+5+1

g(5) = -69

---------

AROC = average rate of change

The AROC of g(x) from x = a to x = b is given by this formula

`\text{AROC} = (g(b)-g(a))/(b-a)\\\\`

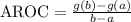
Plug in a = 1 and b = 5. Then simplify.

`\text{AROC} = (g(b)-g(a))/(b-a)\\\\\text{AROC} = (g(5)-g(1))/(5-1)\\\\\text{AROC} = (-69-(-1))/(5-1)\\\\\text{AROC} = (-69+1)/(5-1)\\\\\text{AROC} = (-68)/(4)\\\\\text{AROC} = -17\\\\`

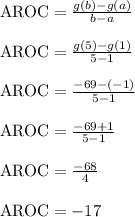
The average rate of change on the interval [1, 5] is -17

This is the same as finding the slope through the lines (1,-1) and (5,-69). Notice the form and structure of the AROC formula mimics the slope formula.

The g(b)-g(a) portion mimics y2-y1 in the numerator.

The b-a portion mimics x2-x1 in the denominator.