Question

The graph represents a function with the form f(x) =asin(bx + c)=Whn10y8-4-O2-X-70-27 -57-47-1-27-23 33qiy27 14:57 2 1 713B-2 4မIV-6+-8+-10+

Answer 1

The standard sine function is given by:

`y=a\sin (bx+c)`

Where:

Amplitude = 2a

Period = 2π/b

Horizontal shift = c

In this case, we have:

- Maximum of 6 and minimum of negative 6, hence the amplitude is 12, this is

`\begin{gathered} 2a=12 \\ (2a)/(2)=(12)/(2) \\ a=6 \end{gathered}`

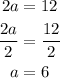
- The period is 2π/3, therefore:

`\begin{gathered} (2\pi)/(3)=(2\pi)/(b) \\ b\cdot2\pi=3\cdot2\pi \\ b\cdot(2\pi)/(2\pi)=3\cdot(2\pi)/(2\pi) \\ b=3 \end{gathered}`

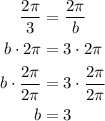
- The graph passes through the y-axis at (0,2), which is 1/3 of the maximum. Considering the shift, we have

`c=\pi`

Answer:

a = 6, b = 3, c = π