Question

How do you know that a solution will be an imaginary number

Answer 1

A second degree equation of the form

`ax^2+bx+c=0`

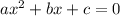
always have solutions given by

`x=\frac{-b\pm\sqrt[]{b^2-4ac}}{2a}`

this is called the general formula. In the general formula we have the number

`b^2-4ac`

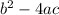
inside the squared root, this number is called the discriminant of the equation and it plays an important role in the type of solutions we'll get.

• if the discriminant is greater than zero then the equation has two real solutions.

,

• If the discrimiant is equal to zero then the equation has a real solution with multiplicity two.

,

• If the discriminant is less than zero then the equation has two complex solutions, one conjugate of the other.

For example, think of the equation

`x^2=-9`

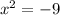
This, in standard form, is written as:

`x^2+9=0`

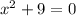
from here we see that a=1, b=0 and c=9. Then,

`\begin{gathered} x=\frac{-0\pm\sqrt[]{0^2-4(1)(9)}}{2(1)} \\ =\frac{\pm\sqrt[]{-36}}{2} \\ =\frac{\pm\sqrt[]{-9}\sqrt[]{4}}{2} \\ =(\pm2\cdot3i)/(2) \\ =\pm3i \end{gathered}`

Notice that in this case the discriminant was less than zero, then we will expect the solutions to be complex numbers.