Question

Consider the line 5x-6y=1What is the slope of a line parallel to this line?What is the slope of a line perpendicular tothis line?

Answer 1

Step-by-step explanation

The equation of a line in slope-intercept form looks like this:

`y=mx+b`

Where m is known as the slope. All lines that have the same slope m are parallel whereas a line perpendicular to y=mx+b has a slope given by -1/m.

In this case we have the line 5x-6y=1. We should write it in slope-intercept form. In order to do this we can add 6y to both sides and substract 1 from both sides:

`\begin{gathered} 5x-6y+6y-1=1+6y-1 \\ 5x-1=6y \end{gathered}`

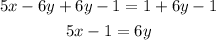
Then we divide both sides by 6:

`\begin{gathered} (6y)/(6)=(5x-1)/(6) \\ y=(5)/(6)x-(1)/(6) \end{gathered}`

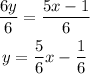
Then the slope of this line is 5/6.

Answer

Following what we stated above we have these answers:

The slope of a line parallel to this is 5/6.

The slope of a line perpendicular to this is -6/5.