Question

If an electron has a kinetic energy of 4.886 MeV, could the classical expression for kinetic energy be used to compute its speed accurately? Support your answer by calculating the true speed of the electron as a fraction of the speed of light.

Answer 1

For this, let's assume that classical expression for kinetic energy will work.

4.886 MeV = 7.8282347 x 10^-13 joules

7.8282347x10^-13 = 1/2(9.1093837 × 10-31)(v^2)

v = 1.311 x 10^9 m/s

The speed of light is 3 x 10^8

This means the electron should have been traveling faster than the speed of light, which is not possible. Classical Expression can't compute speed accurately.

For this we will need to use the relativistic equation

`K=\frac{mc^2}{\sqrt{1-(v^2)/(c^2)}}-mc^2`

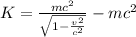
Where K is kinetic energy

Plugging in the numbers

7.828 x 10^-13 = (9.109 × 10-31)(3x10^8)^2/sqrt(1-(v^2)/(3x10^8^2)) - (9.109 × 10-31)(3x10^8)^2

Add the sides

(9.109 × 10-31)(3x10^8)^2/sqrt(1-(v^2)/(3x10^8^2)) = 8.65 x 10 ^-13

multiply by recripercal

(9.109 × 10-31)(3x10^8)^2/8.65 x 10 ^-13 = sqrt(1-(v^2)/(3x10^8^2))

.09477 = sqrt(1-(v^2)/(3x10^8^2))

Square both sides

0.00898 = 1-(v^2)/(3x10^8^2)

subtract the 1

(v^2)/(3x10^8^2) = 1-0.00898

(v^2)/(3x10^8^2) = .99101

multiply by denominator

v^2 = .99101*(3x10^8^2)

v^2 = 8.919x10^16

square root

v = 2.98 x 10^8 m/s

Convert to fraction of speed of light

v = 0.996c