Question

How would you prepare 1.00 L of a 0.400M solution of copper(II)sulfate, CuSO4?

Answer 1

Answer: To prepare the solution of given molarity, we add 63.8 g of copper (II) sulfate to the solution.

Step-by-step explanation:

To calculate the number of moles for given molarity, we use the equation:

`\text{Molarity of the solution}=\frac{\text{Moles of solute}}{\text{Volume of solution (in L)}}`

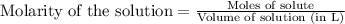
Molarity of copper(II)sulfate solution = 0.400 M

Volume of solution = 1.00 L

Putting values in above equation, we get:

`0.400M=\frac{\text{Moles of copper(II)sulfate}}{1.00L}\\\\\text{Moles of copper(II)sulfate}=(0.400mol/L* 1.00L)=0.400mol`

To calculate the mass of copper(II)sulfate for given number of moles, we use the equation:

`\text{Number of moles}=\frac{\text{Given mass}}{\text{Molar mass}}`

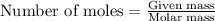
Molar mass of copper(II)sulfate = 159.61 g/mol

Moles of copper(II)sulfate = 0.400 moles

Putting values in above equation, we get:

`0.400mol=\frac{\text{Mass of copper (II) sulfate}}{159.61g/mol}\\\\\text{Mass of copper (II) sulfate}=(0.400mol* 159.61g/mol)=63.8g`

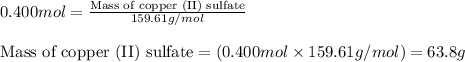
Hence, to prepare the solution of given molarity, we add 63.8 g of copper (II) sulfate to the solution.

Answer 2

We need to dilute 0.400 mol of copper (II) sulfate, how do we know, how many weigh of
`CuSO_4` we have to dilute??

It's simple.


`\eta=(m)/(MM)`

Using a periodic table we can find the molar mass of
`Cu,~~S~~and~~O`


`MM_(CuSO_4)=153.9~g/mol`

Then


`m=\eta*MM`

now we can replace it


`m=0.400*159.6`


`\boxed{\boxed{m=63.84~g}}`

Then we have to dilute 63.84 grams of copper (II) sulfate in 1 L of water to obtain a solution with 0.400M