Question

Use the functions f(x) = 5x − 12 and g(x) = 11x + 2 to complete the function operations listed below. Part A: Find (f +

g. (x). Show your work. (3 points) Part B: Find (f ⋅
g. (x). Show your work. (3 points) Part C: Find f[g(x)]. Show your work. (4 points)

Answer 1

`(f+g)(x)=f(x)+g(x)\\ (f\cdot g)(x)=f(x)\cdot g(x)\\\\ A.\\ (f+g)(x)=5x-12+11x+2=16x-10\\\\ B.\\ (f\cdot g)(x)=(5x-12)(11x+2)\\ (f\cdot g)(x)=55x^2+10x-132x-24\\ (f\cdot g)(x)=55x^2-122x-24\\\\ C.\\ f(g(x))=5(11x+2)-12=55x+10-12=55x-2`

Answer 2

Answer: The completed function operations are :

`(f+g)(x)=16x-10,\\\\(f.g)(x)=55x^2-122x-24,\\\\f[g(x)]=55x-2.`

Step-by-step explanation: We are given two functions as follows :

`f(x)=5x-12,\\\\g(x)=11x+2.`

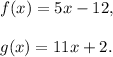
We are to complete the following function operations.

Part A : (f + g)(x)

We have

`(f+g)(x)\\\\=f(x)+g(x)\\\\=(5x-12)+(11x+2)\\\\=16x-10.`

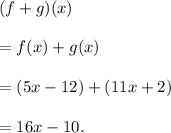
Part B : (f . g)(x)

We have

`(f.g)(x)\\\\=f(x).g(x)\\\\=(5x-12).(11x+2)\\\\=55x^2+10x-132x-24\\\\=55x^2-122x-24.`

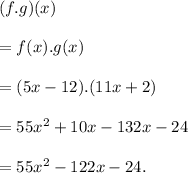
Part C : f[g(x)]

We have

`f[g(x)]\\\\=f(11x+2)\\\\=5(11x+2)-12\\\\=55x+10-12\\\\=55x-2.`

Thus, the completed function operations are :

`(f+g)(x)=16x-10,\\\\(f.g)(x)=55x^2-122x-24,\\\\f[g(x)]=55x-2.`