The area of a circle:
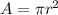
r - the radius, which is equal to half the diameter d
The first circle:

The second circle:
the second circle has a diameter that is four times the diameter of the first circle.
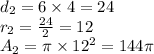
The ratio of the area of the smaller cirlce to the area of the larger circle:
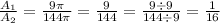
The ratio is 1:16.