Question

Before embarking on a flight, the pilot of a private airplane plans the best route to the final

destination. Suppose the pilot determines one route covers a distance of
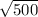
`\sqrt{500` miles and another covers a distance of [tex] \sqrt{605} miles

a. a. Write an expression to represent the difference between the two distances of
each route. Then simplify the expression, keeping your answer in radical form.
Show all steps needed to write your answer in simplest form.

b.b. Use a calculator to change the answer to decimal form. Round the answer to the
nearest tenth. Using this decimal number, write a sentence to explain the
meaning of the answer in this situation.

Answer 1

Differ of these distances:


`√(605) - √(500)`

Simplify this distance:


`√(605) - √(500) = √(121 \cdot 5) - √(100 \cdot 5)= √(121) \cdot √(5)-√(100) \cdot √(5)= \\ \\ = 11√(5)-10√(5)=√(5)`

To a decimal form:
`√(5) \approx 2.2`

Meaning of the answer? The answers 2.2 or
`√(5)` is just very clear to us