Question

1Let f(x) =- 6 (and g(x)9+ 6.22 – 6(fog)(x) =(gof)(x) =

Answer 1

`\begin{gathered} (f\circ g)(x)\text{ }=(x)/(9) \\ (g\circ f)(x)=9x-48 \end{gathered}`

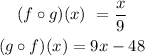
Step-by-step explanation

A composite function is a function that is written inside another function. Composition of a function is done by substituting one function into another function.

f [g (x)] is the composite function of f (x) and g (x). so

`\begin{gathered} f(x)\text{ }=(1)/(x-6) \\ g(x)=(9)/(x)+6 \end{gathered}`

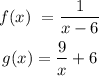
hence

Step 1

`(f\circ g)(x)`

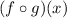
so

`\begin{gathered} f(x)\text{ }=(1)/(x-6) \\ (f\circ g)(x)\text{ }=(1)/(g(x)-6)=(1)/(((9)/(x)+6)-6)=(1)/((9)/(x))=(x)/(9) \\ (f\circ g)(x)\text{ }=(x)/(9) \end{gathered}`

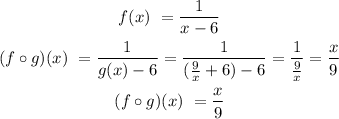
Step 2

`\begin{gathered} (g\circ f)(x) \\ so \\ (g\circ f)(x)=(9)/(f(x))+6=(9)/((1)/(x-6))+6=(9(x-6))/(1)+6=9x-54+6=9x-48 \\ (g\circ f)(x)=9x-48 \end{gathered}`

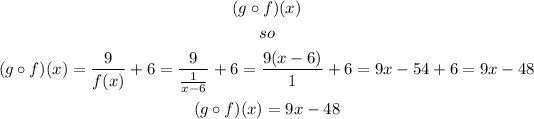
I hope this helps you