Question

Solve the system of linear equations by substitution y=3x-1 and y=x-7

Answer 1

We have the system of equations:

`\begin{gathered} y=3x-1 \\ y=x-7 \end{gathered}`

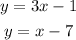
Since we have two equations that are equal to y, we can set them equal to each other.

`3x-1=x-7`

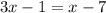
Lets add 1 to both sides of the equation.

`3x=x-6`

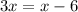
Subtract x from both sides of the equation.

`2x=-6`

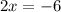
Divide both sides of the equation by 2.

`x=-3`

Now, we can substitute x = -3 back into either equation in order to find its corresponding y-value.

`y=3(-3)-1`

Simplify this equation...

`\begin{gathered} y=-9-1 \\ y=-10 \end{gathered}`

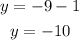
The solution to this system of linear equations is (-3, -10).