Question

The width of a rectangle is 7 meters greater than its length. If the area of the rectangle is 170 square meters, write the quadratic equation in standard form for the equation that would represent the area of the rectangle. Let x equal the length of the rectangle.

Answer 1

It would be (x)(x+7)=170
If you want it solved, then you would get:

`x^(2) +7x-170`=0
(x+17)(x-10)=0
And since you can't have a negative length (-17), x=10
So your side lengths would be 10 and 17 (10+7), which would give you an area of 170.
Hope that helps.

Answer 2

Explanation:

Let the length of the rectangle be=x, then the width of he rectangle will be=7+x.

Also, we are given that the area of the rectangle is equal to=170 square meters, therefore

Area of rectangle=Length×Width

⇒
`170=x(x+7)`

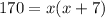
On solving the above equation, we get

⇒
`170=7x+x^2`

⇒
`x^2+7x-170=0`

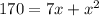
which is the required quadratic equation.

⇒
`x^2+17x-10x-170=0`

⇒⇒
`x(x+17)-10(x+17)=0`

⇒
`(x+17)(x-10)=0`

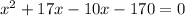
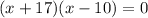
Since, he length of the rectangle cannot be negative, therefore the length of the rectangle=x=10 m

And, the width is=x+7=10+7=17meters.