Question

An 85-kg skier starts from rest at the top of a hill of height 10 m.(a) What will his speed be at the bottom of the hill? Assume the friction between his skies and the snow is negligible and the work done by the force of air resistance is 5.0x103 J.(b) After the skier had reached the bottom of the hill he continues sliding on the horizontal surface there. Another skier, coming from a direction at 90o with the first one direction of motion collides with him. The second skier is 52 kg and his speed before collision is 4.2 m/s. If after the collision they stay entangled together, find their speed and direction. The solution must include a figure showing the velocities of the two objects before and after the collision and a well defined coordinate system.

Answer 1

Step-by-step explanation:

Part a)

By the conservation of energy, we can write the following equation

`\begin{gathered} U_i-W=K_f \\ mgh-W=(1)/(2)mv_f^2 \end{gathered}`

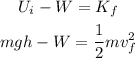
Where m is mass, g is the gravity, h is the height, W is the work done by the force of air resistance, and Vf is the speed at the bottom of the hill.

Replacing m = 85 kg, g = 9.8 m/s², h = 10 m, W = 5 x 10³ J, and solving for Vf, we get

`\begin{gathered} (85\text{ kg\rparen\lparen9.8 m/s}^2)(10\text{ m\rparen-\lparen5}*10^3J)=(1)/(2)(85\text{ kg\rparen v}_f^2 \\ 8330J-5000J=(42.5\text{ kg\rparen v}_f^2 \\ 3330J=(42.5\text{ kg\rparen v}_f^2 \\ \\ \frac{3330\text{ J}}{42.5\text{ kg}}=v_f^2 \\ \\ 78.35=v_f^2 \\ √(78.35)=v_f \\ 8.85\text{ m/s = v}_f \end{gathered}`

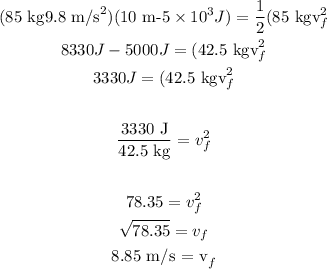
Therefore, the speed at the bottom of the hill is 8.85 m/s

Part b)

We can represent the situation with the following figure

Then, by the conservation of momentum on the x and y-direcction, we can write the following equation

`\begin{gathered} p_(ix)=p_(fx) \\ p_(iy)=p_(fy) \\ \\ m_1v_(1x)=(m_1+m_2)v_(fx)\rightarrow v_(fx)=(m_1v_(1x))/(m_1+m_2) \\ \\ m_2v_(2y)=(m_1+m_2)v_(fy)\rightarrow v_(fy)=(m_2v_(2y))/(m_1+m_2) \end{gathered}`

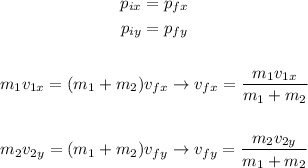
So, we can calculate the final speed on each direction replacing m1 = 85 kg, v1x = 8.85 m/s, m2 = 52 kg, v2y = 4.2 m/s as follows

`\begin{gathered} v_(fx)=\frac{(85\text{ kg\rparen\lparen8.85 m/s\rparen}}{85\text{ kg + 52 kg}}=5.49\text{ m/s} \\ \\ v_(fy)=\frac{(52\text{ kg\rparen\lparen4.2 m/s\rparen}}{85\text{ kg + 52 kg}}=1.59\text{ m/s} \end{gathered}`

Then, by the Pythagorean theorem, we get that the speed after the collision is

`\begin{gathered} v_f=\sqrt{(5.49\text{ m/s\rparen}^2+(1.59\text{ m/s\rparen}^2} \\ v_f=5.72\text{ m/s} \end{gathered}`

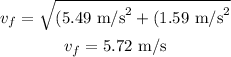
And the direction of the speed is

`\begin{gathered} \theta=\tan^(-1)((v_(fy))/(v_(fx))) \\ \\ \theta=\tan^(-1)((1.59)/(5.49)) \\ \\ \theta=16.15\degree \end{gathered}`

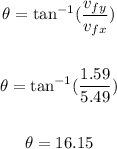
So, the answer is

The speed is 5.72 m/s at a direction of 16.15°