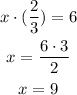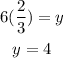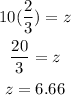Question

Assuming that the shapes are similar. Find the values of x,y, and z

Answer 1

Assuming that both figures have the same shape, we can observe that one is bigger than the other, that means that there is a scale factor that must be found before calculating our values.

We can see that we have one side of both figures have the exact value, we will use these to find the scale factor

`\begin{gathered} X\to\text{scale factor} \\ X=(8)/(12) \\ X=(2)/(3) \end{gathered}`

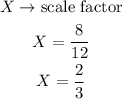
Now we are going to multiply each of the sides of the big figure by the scale factor and we are going to match it with the corresponding side of the small figure.

To prove that the scale is correct, we try it with the known side:

`\begin{gathered} 12X=8 \\ 12((2)/(3))=8\to\text{correct } \end{gathered}`

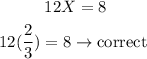
Now we calculate x, y and z

`\begin{gathered} x\cdot((2)/(3))=6 \\ x=(6\cdot3)/(2) \\ x=9 \end{gathered}`
`\begin{gathered} 6((2)/(3))=y \\ y=4 \end{gathered}`
`\begin{gathered} 10((2)/(3))=z \\ (20)/(3)=z \\ z=6.66 \end{gathered}`