Question

How do I do c? I can’t seem to figure these ones out

Answer 1

`\begin{gathered} D=(-\infty,-4)U(-4,5)U(5,\infty) \\ (x^2+2x)/(x^2-9x+20) \end{gathered}`

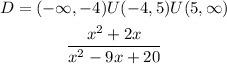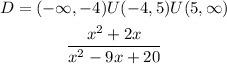
c) For this expression, let's start by rewriting it as a rational function:

`\begin{gathered} y=(x)/(x+4)\cdot(x+2)/(x-5) \\ y=\frac{x(x+2)_{}}{(x+4)(x-5)} \end{gathered}`

So to find out the Domain, we need to determine for which values do this function is defined. So looking at the denominator we can write out:

`\begin{gathered} x+4\\e0 \\ x\\e-4 \\ x-5\\e0 \\ x\\e5 \\ D=(-\infty,-4)U(-4,5)U(5,\infty) \end{gathered}`

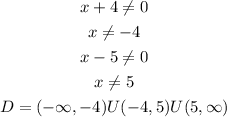
Note that if we plug into the denominator x=5 or x=-4 the function becomes undefined for the denominator would become equal to 0. For all values but -4, and 5 this function is defined.

We could draw the diagram to check the intersections.

2) Now, let's perform the product of that ratios, as indicated.

`(x)/(x-4)\cdot(x+2)/(x-5)=(x(x+2))/((x-4)(x-5))=(x^2+2x)/(x^2-5x-4x+20)=(x^2+2x)/(x^2-9x+20)`

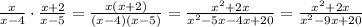
Note that when we multiply ratios we multiply both numerator and denominator simultaneously. We can leave it in its factored form as well.

3) Hence, the answer is:

`\begin{gathered} D=(-\infty,-4)U(-4,5)U(5,\infty) \\ (x^2+2x)/(x^2-9x+20) \end{gathered}`