Question

Three tennis balls, each with a diameter of 2.5 inches, are placed inside a cylindrical can with a diameter of 2.7 inches and a height of 8.5 inches. Approximately how much free space is left in the can? Use the formulas Below

Vc=3.14r^2h
Vs=4*3.14r^3/3

Answer 1

Find the volume of the can and subtract the volume of the balls to find the free space.

Using 3.14 for pi:
We know that the area of a cylinder (can) is
`V_c=3.14r^2h`.
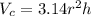
Our diameter is 2.7, so our radius will be half of that, 1.35.
Our height is 8.5.
Let's find the volume.


`3.14*1.35*8.5=36.0315`

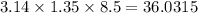
Now, we know that the volume of a sphere (tennis ball) is
`V=\frac{4}33.14r^3`.
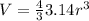
Our diameter is 2.5, so our radius will be half of that, 1.25.
Let's find our volume.


`\frac{4}3*3.14*1.25^3\approx8.17708333`

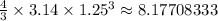
We have three tennis balls, and 3 times that is 24.53125.

Now we subtract the 3 spheres' volumes from our cylinder's volume to find the empty space.

36.0315 - 24.53125 = 11.50025 in³