The value of LP and BL is 6 and 9 respectively. Option A is the right choice.
Since BP = 3, we can write BL = 3k for some positive constant k.
Then by the Centroid Theorem, AP = 2/3 BL=2k.
But we also know that AP =
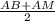
Since AB=AM, we have AP=AB=AM. Therefore, AB = AM = 2k.
Then by the Pythagorean Theorem,
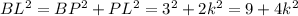
Substituting BL=3k, we get
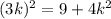
which simplifies to k= 3 / 4.
Hence, BL= 9 and LP = 6.
Final answer in 30 words:
BL=9, LP=6
Option A is the right choice.