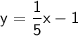Question

I have no idea how to do this: Slope= -3, y-intercept= -1 its slope-intercept form

Slope= -2, y-intercept= -4

Through: (-3,2) , slope=-1/3

Through: (3,2) and (0,-5)

x-5y-5=0

Answer 1

Slope-intercept form is
`\sf~y=mx+b`, where
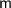
`\sf~m` is the slope and
`\sf~b` is the y-intercept.

Slope = -3, y-intercept = -1

Plug in what we know:


`\sf~y=mx+b`


`\sf~y=-3x-1`

Slope = -2, y-intercept = -4

Plug in what we know:


`\sf~y=mx+b`


`\sf~y=-2x-4`

Through: (-3,2) , slope=-1/3

Okay, here we use point-slope form, and the simplify to get it in slope-intercept form.


`\sf~y-y_1=m(x-x_1)`

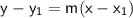
Where
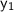
`\sf~y_1` is the y-value of the point,
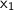
`\sf~x_1` is the x-value of the point, and
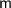
`\sf~m` is the slope.

Plug in what we know:


`\sf~y-2=-(1)/(3)(x+3)`

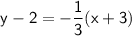
Distribute -1/3 into the parenthesis:


`\sf~y-2=-(1)/(3)x-1`

Add 2 to both sides:


`\sf~y=-(1)/(3)x+1`

Through: (3,2) and (0,-5)

Now we plug this into the slope-formula to find the slope, plug the slope and one of these points into point-slope form, then simplify to get it in slope-intercept form.


`\sf~m=(y_2-y_1)/(x_2-x_1)`

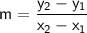
(3, 2), (0, -5)
x1 y1 x2 y2

Plug in what we know:


`\sf~m=(-5-2)/(0-3)`

Subtract:


`\sf~m=(-7)/(-3)`


`\sf~m=(7)/(3)`

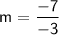
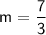
Plug this into point-slope form along with any point.


`\sf~y-y_1=m(x-x_1)`


`\sf~y-2=(7)/(3)(x-3)`

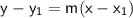
Distribute 7/3 into the parenthesis:


`\sf~y-2=(7)/(3)x-7`

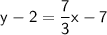
Add 2 to both sides:


`\sf~y=(7)/(3)x-5`

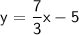
x-5y-5=0

Add 5 to both sides:


`\sf~x-5y=5`

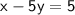
Subtract 'x' to both sides:


`-5y=-x+5`

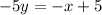
Divide -5 to both sides:


`\sf~y=(1)/(5)x-1`