A line is said to have an x-intercept of -1, and a y-intercept of 4.
It is required to write the equation of the line in standard form.
Recall that the Standard Form of the equation of a line is of the form:
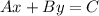
The equation of a line with slope m and y-intercept c in slope-intercept form is:
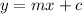
Since the y-intercept is 4, substitute c=4 into the equation:
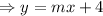
Since the x-intercept is -1, substitute x=-1 and y=0 into the equation to find m:
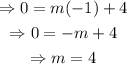
Substitute m=4 back into the equation:
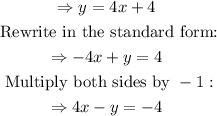
The correct option is a.